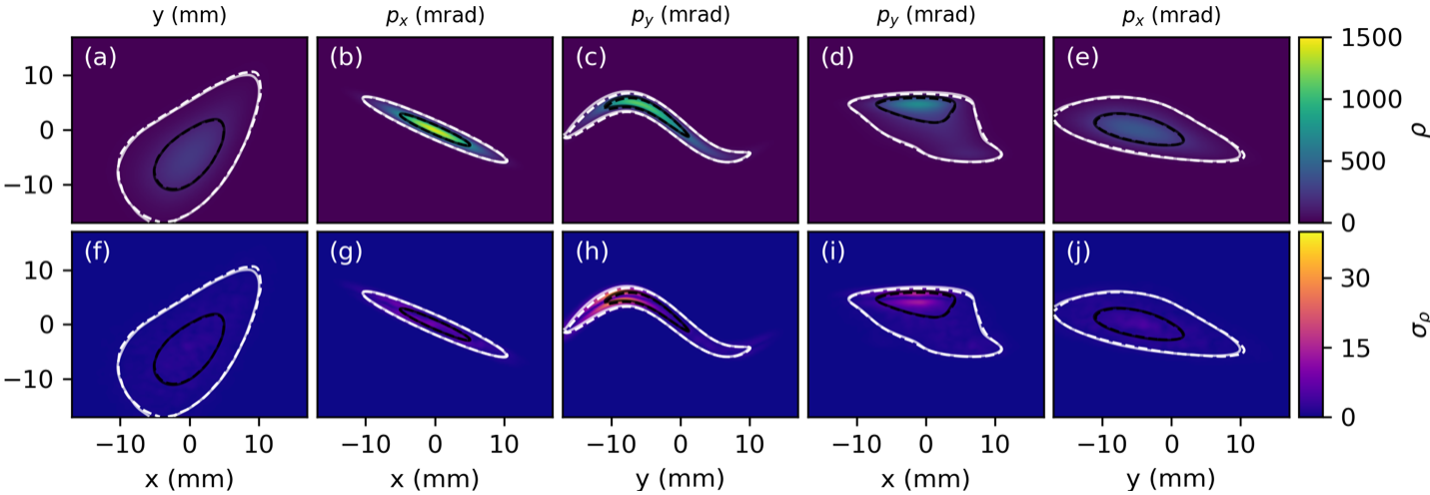
Detailed four-dimensional transverse phase space reconstruction using neural networks and differentiable simulations
Measuring the phase space distribution of particle beams is crucial for understanding beam dynamics in particle accelerators. For instance, novel beam manipulation schemes require detailed high-dimensional measurements of the phase space distribution for effective implementation in experimental setups. However, available high-dimensional phase space reconstruction methods are extremely resource intensive and/or require specialized diagnostic hardware.
In this work, we present a new algorithm that makes use of neural networks and differentiable particle tracking simulations to efficiently reconstruct 4D transverse phase space distributions. The reconstruction only needs a single focusing quadrupole, a diagnostic screen and a limited number of measurements. We demonstrate an unprecedented level of reconstruction detail in both experimental and simulated examples.
This novel reconstruction method is a milestone towards CBB Theme 3 objective of precision phase-space control of particle accelerator systems. This work provides sample efficient, high dimensional phase space reconstructions of beam distributions, enhancing our ability to perform novel beam manipulations that benefit particle accelerator applications.
Reference: